where has all the money gone ?
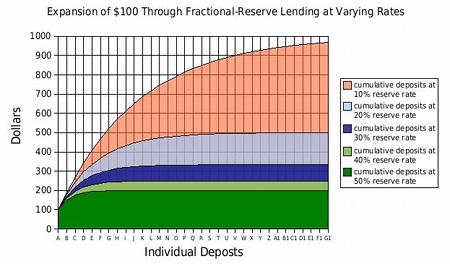
Views on various deposit mountains or the expansion of $100 through fractional-reserve lending at varying rates, under the re-lending model. Each curve approaches a limit. This limit is the value that the money multiplier calculates (diagram from wikipedia)
There is currently quite a debate about what to do with the european financial sector in the Eurozone. The debate is partially a consequence of the European Council outcomes, which are seen as successful by some people, like:
“In his speech at the European Parliament, President Barroso shared his conviction that the European Council and the Euro area summit have delivered results that should strengthen confidence in Europe’s financial stability and pave the way for the future.”
(see http://ec.europa.eu/commission_2010-2014/president/news/index_en.htm and scroll down to “We are in this together and together we will be able to overcome this crisis”), but which are seen problematic by others. So in particular a group of about 160 mostly german economists wrote an open letter which mainly adresses the concept of a EU banking union. The EU banking union (there is currently only a german wikipedia entry on this) is envisaged to include a centralized european control for banks, a common deposit insurance and most important a centralized possibility to recapitalize european banks with money from the ESM. It is this last option which is mostly discussed, like in the letter of the 160 economists it is amongst others pointed out that the debts in the banking sector are three times bigger than the euro countries debts and that the countries with high debts are a majority within the Eurozone and that so the control over the recapitalization schemes is questionable. In the letter the possibility of bank bankruptsies is also discussed (see also this randform post). The critics of the 160 economists where regarded by some politicians as “irresponible” and in the turn other economists critized the letter as “holding questionable arguments and a language which is formed by national cliches” (see Tagesschau).
Unfortuantely in all these discussions I missed the discussions about two really important measures which are first the seperation of retail and investment sector (“Glass-Steagall”), which is astonishingly pushed forward by the british (see e.g. the article on zerohedge) and as it seems less pushed by the US (see e.g. this article by E. Stourton) and secondly the discussion about reserve requirements like within the discussions about Basel III.
I don’t want to speak about the first measure, which I find rather necessary, but about the second measure and the possible role of the reserve ratio in the european banking crisis and the consequences of this role.
Lets briefly explain the essential ingredients of the reserve ratio. A fundamental feature of a typical money lending scheme is that you can lend out allmost all of your money, while keeping only a little share as “security money” (the reserve) for yourself. The amount of money you lend out divided by the original amount of money is simplified called reserve ratio or for the case of banks often also reserve requirement. Sometimes one uses also the inverse of the reserve ratio, a socalled money multiplier.
Imagine a “chain” of lenders (that is one starts with a lender A who gives money to a lender B a.s.o). Each lender keeps lets say ten percent of his/her money and lends out the rest ninety percent to the next in the chain. Wikipedia has a table with such chain starting with 100$ at lender A and ending at 10.74$ at lender K with a 20 percent ratio. Assume each lender does some bookeeping about what had been lent out. At the table one sees that in the books of all the lenders a total amount of 357.05$ is noted as being lent out. This sum is more than three times the original amount of 100$. It should be rather clear that this “lent out”-sum gets bigger if the chain gets longer and/or if the reserve ratio gets smaller. The above diagram (which I haven’t checked) from wikipedia shows how the deposits vary for different money multipliers. Note that the “lent out”- sum is somewhat “virtual” that is it is not the original “real” amount of 100$ dollars, but the sum of what is “missing” in the books. In particular in the case that a borrower can’t pay back his/her debts the lending chain terminates and each bank would ask for that what is claimed as missing in the books, i.e. in the example all lenders together would ask for the amount of 357.05.$ In other words: it is mainly this “lent out” money which is seen as been missing by the banks rather then the “real” money, because each bank or lender sees only its own debts.
If you imagine that a bank is part of many chains of this sort then it becomes clear how rather few failed paybacks at the end of long lending chains can lead to a quite big “missing” sum of money.
So what would you do if you would be a rich donor and would like to help the banks with their debts? Give each bank that share, which is missing in its books? Of course not! Of course in order to save money you would rather try to pay out the “ends of the chains” which allows for inverting the whole lending out process. For the case of the spanish banking crisis (which is mostly in charge for the whole spanish financial crisis) this would for example mean to help people who couldn’t pay back the loans for their appartments because of job losses (like e.g. this case).
By the above it becomes also clear that the concrete amount of the reserve ratio may play a rather big role in the stability of banks. In this context it is instructive to look at the wikipedia table of different countries reserve ratios. It is clear that changing the reserve ratio in this crisis would make everything worse for a lot of banks. Nevertheless it is an issue which should be discussed, especially if one asks the question of where has all the money gone.